CryptoDB
Programmable Distributed Point Functions
| Authors: |
|
|---|---|
| Download: | |
| Conference: | CRYPTO 2022 |
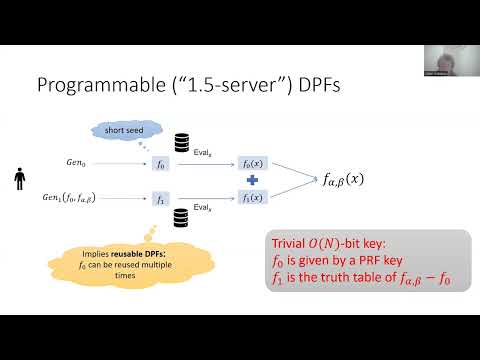
| Abstract: | A distributed point function (DPF) is a cryptographic primitive that enables compressed additive sharing of a secret unit vector across two or more parties. Despite growing ubiquity within applications and notable research efforts, the best 2-party DPF construction to date remains the tree-based construction from (Boyle et al, CCS’16), with no significantly new approaches since. We present a new framework for 2-party DPF construction, which applies in the setting of feasible (polynomial-size) domains. This captures in particular all DPF applications in which the keys are expanded to the full domain. Our approach is motivated by a strengthened notion we put forth, of programmable DPF (PDPF): in which a short, input-independent “offline” key can be reused for sharing many point functions. – PDPF from OWF. We construct a PDPF for feasible domains from the minimal assumption that one-way functions exist, where the second “online” key size is polylogarithmic in the domain size N. Our approach offers multiple new efficiency features and applications: – Privately puncturable PRFs. Our PDPF gives the first OWF-based privately puncturable PRFs (for feasible domains) with sublinear keys. – O(1)-round distributed DPF Gen. We obtain a (standard) DPF with polylog-size keys that admits an analog of Doerner-shelat (CCS’17) distributed key generation, requiring only O(1) rounds (versus log N). – PCG with 1 short key. Compressing useful correlations for secure computation, where one key size is of minimal size. This provides up to exponential communication savings in some application scenarios. |
Video from CRYPTO 2022
BibTeX
@inproceedings{crypto-2022-32253,
title={Programmable Distributed Point Functions},
publisher={Springer-Verlag},
author={Victor I. Kolobov and Elette Boyle and Niv Gilboa and Yuval Ishai},
year=2022
}